Jobs

The multimode quantum optics group pioneered the modal approach to quantum metrology [6] and obtained unparalleled sensitivity in source separation estimation [2] using a unique technology invented in the group [3]. The group has a strong experimental focus, but is also engaged in purely theoretical activities aiming at developing quantum optics in the continuous variable (CV) framework.
 
In a nutshell 

Why to apply:
 -you are passionate about fundamental quantum science and/or technological applications 
 -you will benefit from an international team of researchers who are experts in Continuous Variable quantum optics and metrology (theory and experiment) 
– you will be in a group at the core of many national and international research networks
 
Our specific view:
– Experiments and theory should go together to implement practical parameter estimation at the quantum limit
– Modal approach to quantum metrology allows both for quantum limited parameter estimation and to derive theoretical bounds closely related 

Environment
As a whole, the group has a tradition of working together with a diverse range of people from varied backgrounds. This diversity often leads to fruitful scientific input from different points of view, and it allows the group to explore new avenues. Furthermore, the moderate size of our group gives PhD students and postdocs the opportunity to discuss with PIs on a daily basis. This fosters a dynamic atmosphere with a lot of space for discussion.
 
Your work will be supported by the CEA-Audace project Lumiere, which aims to provide support and build collaborations. Notably, the project will enable you to gain access to groundbreaking multi-photon detectors with very high sensitivity and fast response time, promising significant advancements in metrology and tracking applications.
The project is inherently interdisciplinary, bridging quantum optics with fields such as signal processing, machine learning, and advanced instrumentation. It also benefits from strong collaborations with national and international teams, ensuring frequent exchanges of ideas and expertise. 
 
 
Practical information  
Candidates must hold an internationally recognized PhD in a field related to experimental quantum physics. A good background and past research track record in experimental optics, and quantum physics is required. 
Application procedure: Inquiries and applications should be sent by email to Nicolas Treps(nicolas.treps@lkb.upmc.fr). Applications should include a detailed CV and two names of potential referees
Salary: Monthly net salary (after-tax) between 2200€ and 2900€, depending on experience
Application deadline:  Preferentially apply before 16 May 2025
Starting date: flexible
Duration: 2 years (with possible renewal of 1 year)
 
References
 
[1] Tsang, M., Nair, R., & Lu, X. M. (2016). Quantum theory of superresolution for two incoherent optical point sources. Physical Review X, 6(3), 031033.
[2] Rouvière, C., Barral, D., Grateau, A., Karuseichyk, I., Sorelli, G., Walschaers, M., & Treps, N. (2024). Ultra-sensitive separation estimation of optical sources. Optica, 11(2), 166-170.
[3] G. Labroille, BertrDenolle, P. Jian, P. Genevaux, N. Treps, and J.-F. Morizur, Efficient and mode selective spatial mode multiplexer based on multi-plane light conversion, Opt Express 22, 15599 (2014).
[4] Gessner, M., Treps, N., & Fabre, C. (2023). Estimation of a parameter encoded in the modal structure of a light beam: a quantum theory. Optica, 10(8), 996-999.
[5] G. Sorelli, M. Gessner, M. Walschaers, and N. Treps, Optimal Observables and Estimators for Practical Superresolution Imaging, Phys. Rev. Lett. 127, 123604 (2021).
[6] C. Fabre and N. Treps, Modes and States in Quantum Optics, Rev. Mod. Phys. 92, 035005 (2020).

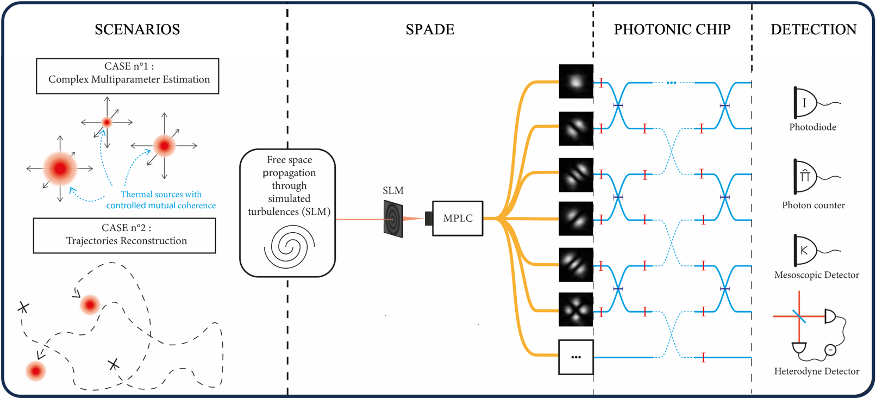
It was long believed that the ultimate resolution limit in imaging was dictated by the Rayleigh criterion, which states that two point sources are indistinguishable when their images overlap excessively. This diffraction limit, often considered a fundamental barrier in conventional imaging systems, posed a significant challenge for resolving closely spaced objects. However, recent advances in quantum metrology have revealed that the Rayleigh limit is not a fundamental boundary [1]. Employing non-conventional imaging techniques, inspired by quantum metrology, it is possible to achieve super-resolution imaging, surpassing the classical resolution limits [2,3]. One such approach is pursued in the PESto experiment at LKB, where Spatial Mode Demultiplexing (SPADE) is used. The light from two point sources is demultiplexed into a basis of Hermite-Gaussian spatial modes. Detecting and counting photons in each spatial mode of the multimode light, the distance between the two point sources is estimated with a precision approaching the quantum limit [4], order of magnitudes better than the Raileigh limit. 
In practical imaging scenarios, multiple parameters must often be estimated simultaneously, making the problem more complex [5]. Notably, the SPADE technique is only quantum-optimal when only one parameter is to be estimated, and the others, such as the centroid of the source distribution, the relative intensity between the sources 0r even the number of sources, are known. This PhD project aims to extend the capabilities of SPADE to more realistic scenarios, incorporating multi-parameter estimation, low-flux detection down to the single photon level, and the effects of environmental factors such as optical turbulence. Addressing these complexities requires the integration of machine-learning techniques to optimize the choice of spatial modes, extract multiple parameters from the data efficiently, and ensure robustness against experimental imperfections. Additionally, in scenarios involving dynamic or moving sources—where only limited information can be gathered in real-time—a Bayesian approach to estimation will be explored to track the sources effectively. 
This research will focus on advancing super-resolution imaging in realistic conditions, providing solutions to the challenges of multi-parameter estimation and developing methods to handle experimental imperfections and source motion. By working on both experiment and theory, leveraging estimation theory -classical and quantum-, machine learning and Bayesian techniques, the goal is to achieve unprecedented imaging precision and pave the way to a new paradigm in imaging.

Contact: Nicolas Treps, nicolas.treps@lkb.upmc.fr
 

[1] Tsang, M., Nair, R., & Lu, X. M. (2016). Quantum theory of superresolution for two incoherent optical point sources. Physical Review X, 6(3), 031033.
[2] Gessner, M., Treps, N., & Fabre, C. (2023). Estimation of a parameter encoded in the modal structure of a light beam: a quantum theory. Optica, 10(8), 996-999.
[3] Sorelli, M. Gessner, M. Walschaers, and N. Treps, Quantum limits for resolving Gaussian sources, Phys. Rev. Research 4, L032022 (2022).
[4] Rouvière, C., Barral, D., Grateau, A., Karuseichyk, I., Sorelli, G., Walschaers, M., & Treps, N. (2024). Ultra-sensitive separation estimation of optical sources. Optica, 11(2), 166-170.
[5] Řehaček, J., Hradil, Z., Stoklasa, B., Paúr, M., Grover, J., Krzic, A., & Sánchez-Soto, L. L. (2017). Multiparameter quantum metrology of incoherent point sources: towards realistic superresolution. Physical Review A, 96(6), 062107.
[6] C. Fabre and N. Treps, Modes and States in Quantum Optics, Rev. Mod. Phys. 92, 035005 (2020).